11
$\begingroup$

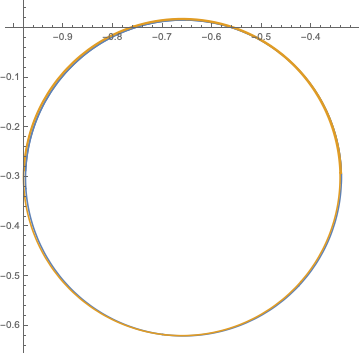
start at the origin, first step number is 1.

  • turn $\pi/n$
  • move $1/n$ units forward

Angles are cumulative, so this procedure is equivalent (finitely) to $$ u(k):=\sum_{n=1}^{k} \frac{\exp(\pi i H_{n})}{n}$$

  • Is the limiting shape formed by a line plot of partial sums a circle or a spiral? Relying on visual intuition with the harmonic numbers seems to be perilous.
  • Where's its center? This is equivalent to "If $u(k)$ converges, what does it converge to?".

Naively resumming within exp produces lots of divergent series as a result of the harmonic numbers $H_{n}$. At the cost of being a numerically ill-conditioned sum with lots of cancellation, I have to wonder what this says of arithmetic properties of the harmonic numbers.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
12
$\begingroup$

The large-$n$ asymptotics of the harmonic numbers is $H_n\simeq \gamma_E+\log n$, which we use for $n\geq k_0$, replacing the sum $\sum_{n=k_{0}}^k$ by an integral $\int_{k_0}^k dn$. We thus find $$\begin{align} U(k)&=u(k_0-1)+e^{i\pi\gamma_E}\int_{k_0}^k \frac{1}{n}e^{i\pi\log n}\,dn\\ &=u(k_0-1)+\frac{i}{\pi} e^{i \pi\gamma_E } \left(k_0^{i\pi}-k^{i \pi }\right). \end{align}$$ So $U(k)$ traces out a circle in the complex plane, of radius $1/\pi$ and center at $z_0=u(k_0-1)+(i/\pi)e^{i \pi\gamma_E } k_0^{i\pi}$.

The plot compares $u(k)$ (gold) and $U(k)$ (blue) for $k_0=50$ and $k$ up to 1000, when $z_0=-0.66- 0.28i$. The agreement is quite satisfactory.

Improve this answer
$\endgroup$
5
  • 4
    $\begingroup$ While this suggests that $u(k)$ also converges to a circle of radius $1/\pi$, the centers of the two circles will be unrelated. $\endgroup$
    – Emil Jeřábek
    Aug 14, 2019 at 11:05
  • 3
    $\begingroup$ The plot seems to confirm the value of the radius but not of the coordinates of the center. $\endgroup$
    – Sylvain JULIEN
    Aug 14, 2019 at 11:10
  • 1
    $\begingroup$ Using H_n = \log{n} + \gamma + O(1/n), \exp(x + \eps) = \exp(x) (1 + O(\eps)), and partial summation (in the form \int_1^X t^{-1+\pi i} d\floor{t} = \int_1^X t^{-1+\pi i} dt + O(\int_1^X \{t\} t^{-2+\pi i} dt) ), I believe this answer does indeed give an asymptotic (and explains the offset of the centre mentioned above). $\endgroup$
    – alpoge
    Aug 14, 2019 at 11:34
  • $\begingroup$ fixed the center offset. $\endgroup$
    – Carlo Beenakker
    Aug 14, 2019 at 11:59
  • 2
    $\begingroup$ The comment by @alpoge may be more easily read as Using $H_n = \log{n} + \gamma + O(1/n)$, $\exp(x + \epsilon) = \exp(x) (1 + O(\epsilon))$, and partial summation (in the form $\int_1^X t^{-1+\pi i} d\lfloor{t}\rfloor = \int_1^X t^{-1+\pi i} dt + O(\int_1^X \{t\} t^{-2+\pi i} dt)$ ), I believe this answer does indeed give an asymptotic (and explains the offset of the centre mentioned above). $\endgroup$
    – Gerry Myerson
    Aug 14, 2019 at 13:07

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge that you have read and understand our privacy policy and code of conduct.