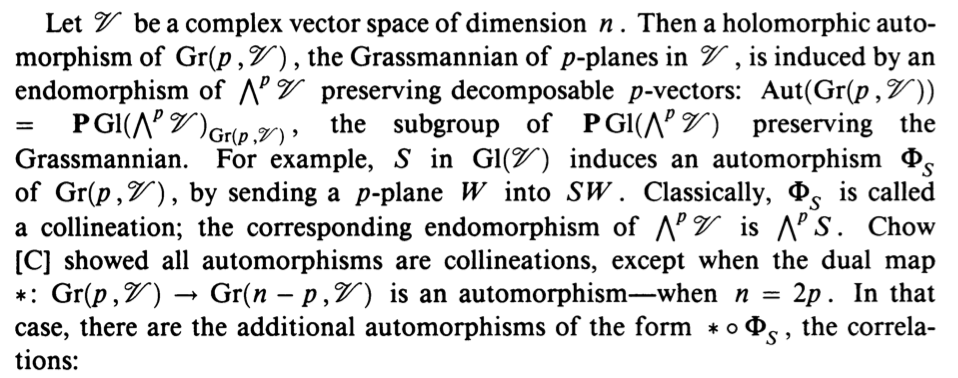
I want to know what are the holomorphic automorphisms of a Grassmannian. Can someone tell me this?
$\begingroup$
$\endgroup$
3
-
2$\begingroup$ ams.org/journals/proc/1989-106-01/S0002-9939-1989-0938909-8 $\endgroup$– Carlo BeenakkerJul 1, 2014 at 6:29
-
1$\begingroup$ @Carlo: I suggest that you promote your comment to an answer. $\endgroup$– Neil StricklandJul 1, 2014 at 8:17
-
5$\begingroup$ It probably would have been a good idea to quote the paper by Wei-Liang Chow, On the geometry of algebraic homogeneous spaces, Ann. of Math. 50 (1949), 32–67, since that is where the original argument is made. $\endgroup$– Robert BryantJul 1, 2014 at 16:17
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
4
Automorphisms of Grassmannians, Michael J. Cowen (1989).
answered Jul 1, 2014 at 8:35
-
-
$\begingroup$ @Mostafa -- see section 2 of Cowen's paper for the construction of the dual map. $\endgroup$ Jul 1, 2014 at 9:51
-
6$\begingroup$ Demazure also gave a complete characterization of automorphism groups of generalized Grassmannians $G/P$, "Automorphismes et deformations des varietes de Borel", Invent. Math. 1977. Essentially, the automorphism group of $G/P$ is generated by $G$ (adjoint form) together with certain automorphisms of the Dynkin diagram. The above is a special case, since $Gr(k,n) = PGL_n/P$. $\endgroup$ Jul 1, 2014 at 14:02
-
$\begingroup$ @CarloBeenakker: However, when $n=2p=2$, our Grassmannian ${\rm Gr}(1,{\Bbb C}^2)$ is the projective line ${\Bbb P}^1$, and its automorphism group is ${\rm PGL}(2,{\Bbb C})$ without additional automorphisms, contrary to what Cowen writes. $\endgroup$ Sep 2 at 12:43